Introduction
Ever look at a wire fence, or something with a pattern like the ones above? Ever notice the weird pattern that you get when you are looking through two of them?
I’m going to try to explain that here (the general theory comes up in many, many different fields).
Abstraction
The first step to explaining this phenomenon is to examine a slightly more abstract case. (awful I know, but it.s short and has to be done now).
Consider a 1 dimensonal periodic wave which the following properties:

This represents as boring and regular a periodic function as you could imagine:

That.s pretty simple yeah? Cool.
Right, now, lets consider what happens if you take two of these functions, and increase the period of one of them slightly as follows:
![]()
Now just have to overlap this one with the original one (if the area of either is black, the so is the resulting area, and conversely an area is only white if both areas are white). The following function is the result:
Now, because both of the wavelengths of the functions inside are quotient numbers, the above function is also periodic, and is equal to the lowest common integer multiple of the wavelengths. Call this resultant wavelength L.
Here’s an example of the overlapping of two simple waves:
![]()
Notice how the brightest patch appears on the area of maximum overlap, and how the dark areas coincide with areas where there’s so little overlap that very little white remains, or equivalently, where there’s so much interference so that very little white remains, or equivalently where the waves are out of sync.
Keep this in mind.
3-D Example
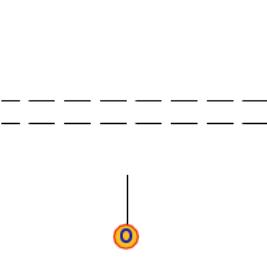
Looking at the above illustration, in which an observer, when faced with two parallel, waves as shown, as one would expects, observes them. Due to the simple effect of perspective, the wave further away appears smaller than the one closer, so what the observer observes is pretty much the same as what one sees with the Φ function.
The following diagram shows the areas of whiteness that the observer sees enclosed by red lines, while the areas of black shown by some pink ones cut off.
Now, let.s look in a bit more detail at what he sees in a more general case.
Given that the waves are not in phase, so that the area of most whiteness doesn’t occur straight in front of the observer.
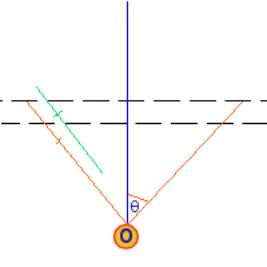
The interference betweeen the two waves at a maximum at Θ=Θ1 and Θ=-Θ1 and at an infinite number of other greater angles (because it is equivalent to the %Phi; function, white patches occur periodically).
Notice the aquamarine line parallel to the red line on the left; this also shows a line of maximum whiteness. Lines parallel to this one will always represent an area of maximum whiteness, because it they are the lines that join areas of one wave to an identical area of the other. Even if a line intersects an area in which there is nothing but blackness, this is still an area of maximum whiteness as the nearest white areas will have as much overlap as they can.
If the observer moves parallel to the plates, then the previous line that represented the angle of maximum interference (and therefore brightness) no longer intersects the observer so one must choose a line of sight that does.
Now it should be obvious that all the lines which represent the areas of maximum whiteness will all be parallel to the lines which did so when it was in it’s original position. Therefore the observed Φ function does not change at all with parallel transformation of the observer.
Now, should the observer move perpendicular to the waves, one can similarly keep the same relative lines which represent maximum whiteness, because they’ll be parallel to the original ones and therefore totally valid.
Therefore, the observed Φ function does not change with perpendicular transformations of the observer.
Therefore, the observed Φ function is constant regardless of the position of the observer.
Solution to the grating problem
Now, what happens if the Ψ function is 2-dimensional?
Well, that depends on its symmetries. Let.s have a look at some examples:

Now wherever a point of maximum whiteness is, there’s also another one along one of the lines of symmetry (the pattern formed along the lines of symmetry because of parallel overlap is pretty much the same as the Φ function). One of the three symmetry flows from the above pattern is shown below:

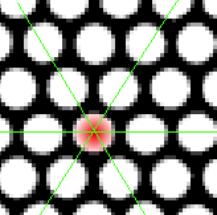
Now, look at the diagram below, with an area of maximum whiteness represented by the red dot.

Now from this point we can draw three lines of symmetry:
And lets just say for simplicity that the pattern repeats ever 2 spaces. Following the horizontal line we can find some other white spaces..
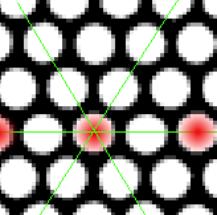
and similarly we can follow the other lines around to generate the others (I.ve zoomed out a bit and haven.t depicted the underlying pattern.but that doesn.t matter much anyway)
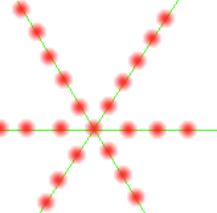
But from each of these white patches additional lines of symmetry can be formed so that you end up with the following:
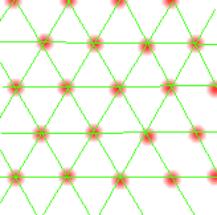
see!!!! The apparent pattern mirrors the underlying pattern structure :) (though it’s not an exact duplicate naturally). The spaces in between are blacker because less interference occurs in those. (the exact pattern isn.t really that important and can be worked out for simple patterns pretty easily but I might as well stick to the general case)
Because of what I said in section 3 this image always stays in the same position relative to you, so if you move towards it the pattern gets smaller relative to it’s surroundings but it takes up the same amount of what we see, and also the symmetry of the intereference pattern is the direct intersection of the symmetries of the underlying patterns.
Anyway that’s about it, hope it explains the interference patterns formed by wire fences and perforated screens :)